Distance of the Horizon

Given the Height of Eye, Compute the Distance to the Horizon

Now, how would you figure out the solution to this problem - one which could become of great importance in the real world!!

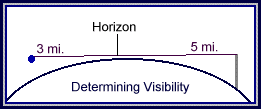
Well, let's assume you were the one on the left. Your 'distance to the horizon' was computed to be 3 miles after you plugged your height above water into the formula on this page [or from other modalities]. You see a light in the distance, and for reasons not explained on this page, you know it is that of another boat.  Yes, you see this light right 'on' the horizon, 'touching' the Earth' sphere.  Now you want to use your VHF radio to call him. Will you get him?
OK, this is a trick question, in part, but also has some important points to consider.  First, does the other person have his radio on?  Well, we obviously are hoping he does as our boat is sinking. Then, how far will our radio transmit? 
We'll take that up on another web page, but for purposes of making this question more difficult, let's say that we cannot depend on the line-of-sight rule, but instead have to do some figuring.  Well, though the above diagram says '5 miles', we wouldn't know that, unless we were speaking to the other guy already and was told that information. Thus, how can we figure that out? Some common sense comes into play here, and some guesstimating.  And, having this web site on your boat to help you do some figuring may come in handy.  We need to know how tall that boat (light) is, but we don't. So, we'll guess. Obviously we can figure out that it can be either shorter boat [in height] that is closer to us, or a taller boat. But how tall. Well, unless it's an ocean liner [in Jamaica bay??], we can guess that the tallest thing we may see is 38 feet. (where did I come up with that? Last boat I was on needed that height to clear the bridges. Pick another number to be even more realistic, and safe). So, believe it or not if you plug '38' into the above formula, you get only about 8+ miles. No, you probably don't want to swim it, but, if we ADD THE 8 MILES TO THE 3 THAT WE KNOW FOR US, we get 11. And we should know whether or not our VHF radio can handle that.

That was easy. In reality, we most often would be working with 'one side' of the above diagram. If we don't see it coming over the other side of the Earth [horizon], then we can assume it is WITHIN the 3 miles. Probably less.

Here is a very practical and good homework assignment. When we look at charts (and we all should have one aboard, a real one, not just an electronic image on a GPS. They fail, you know.) you will note that they will indicate the height above water/land of certain structures. Perhaps a water tank that is near the shoreline. You can use this knowledge to figure out how far you are from there. Send me your answers.

Other Conversions and Calculations